Research on near/far-field flow characteristics of caved ore and rock based on rigid block model
-
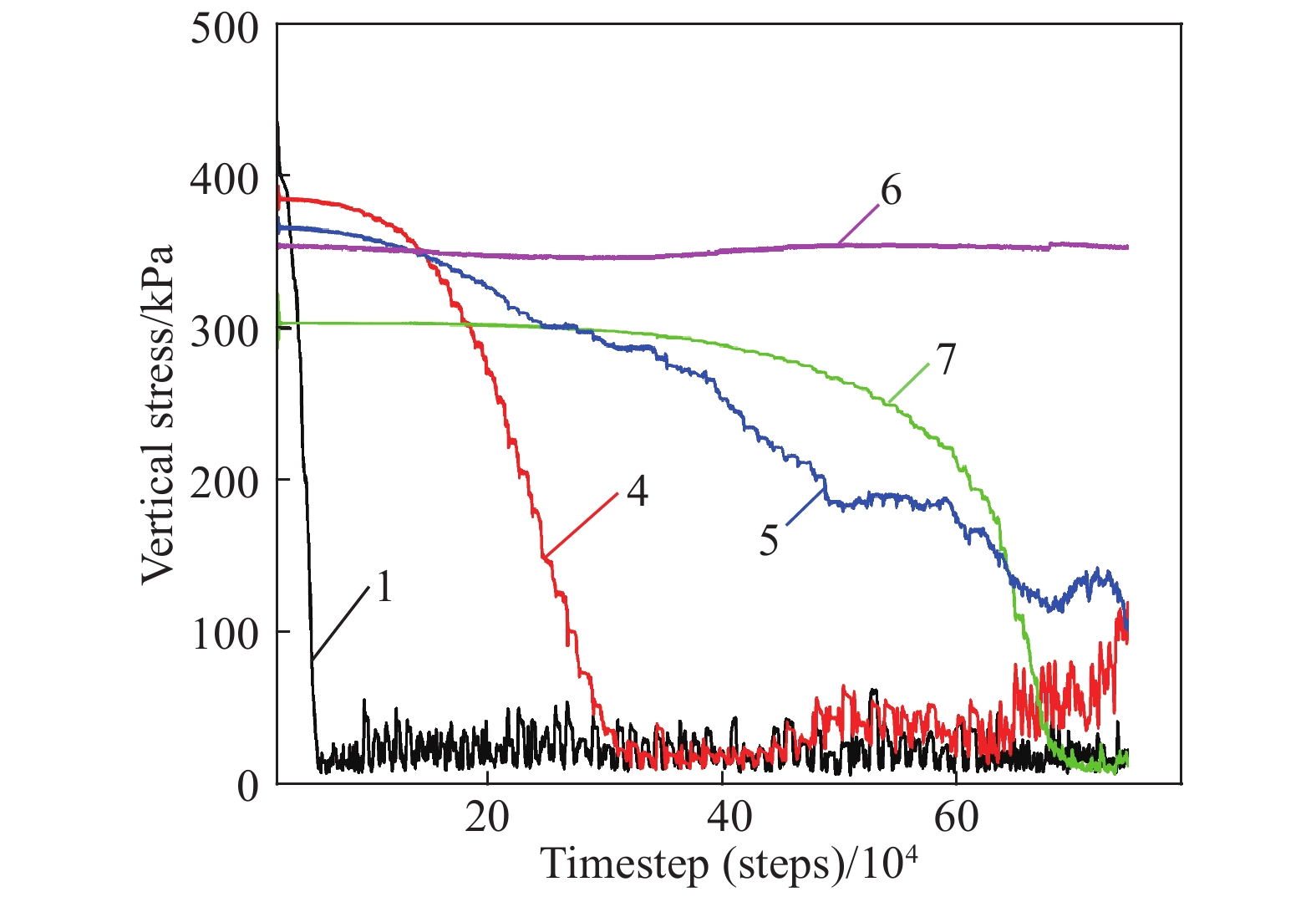
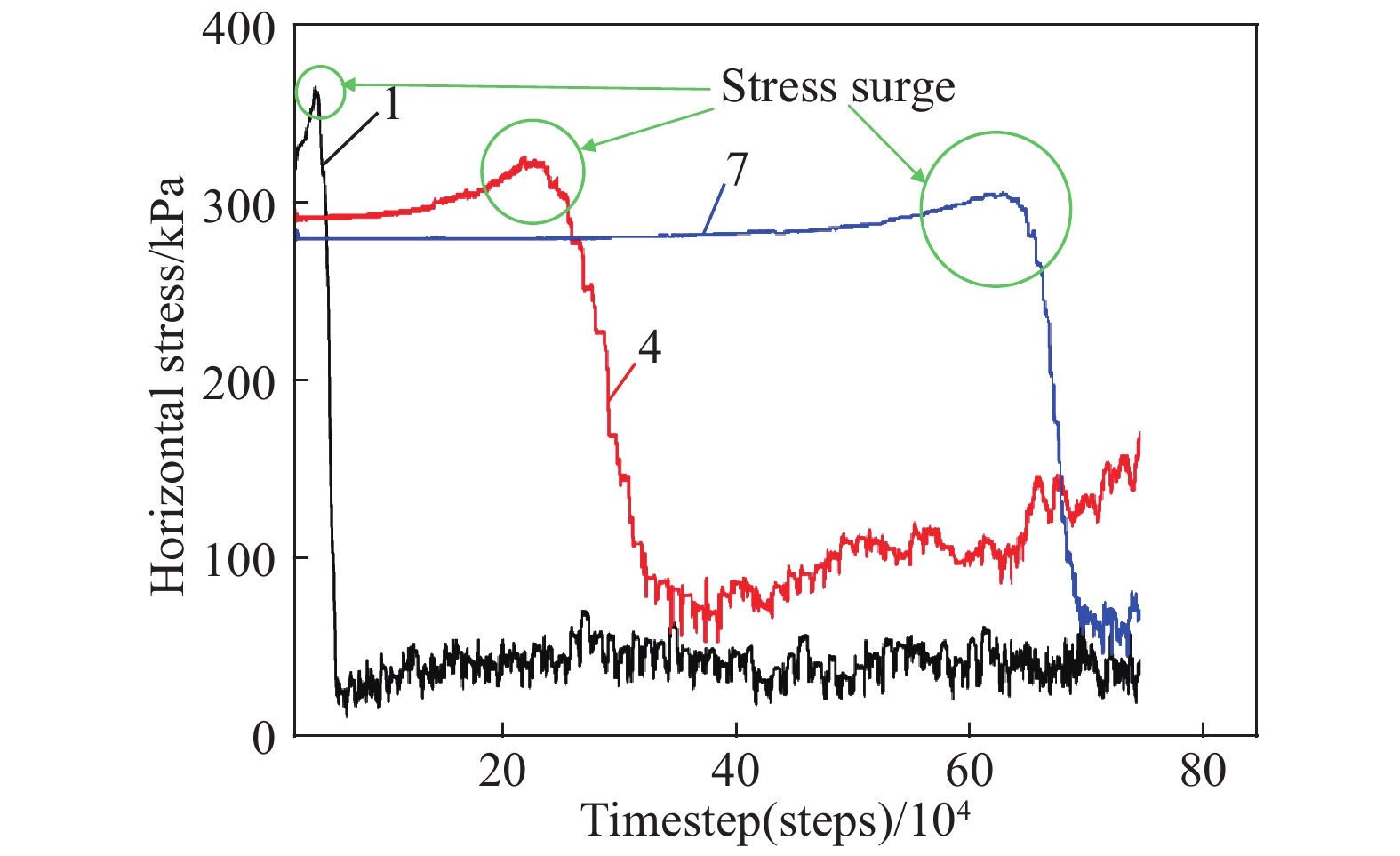
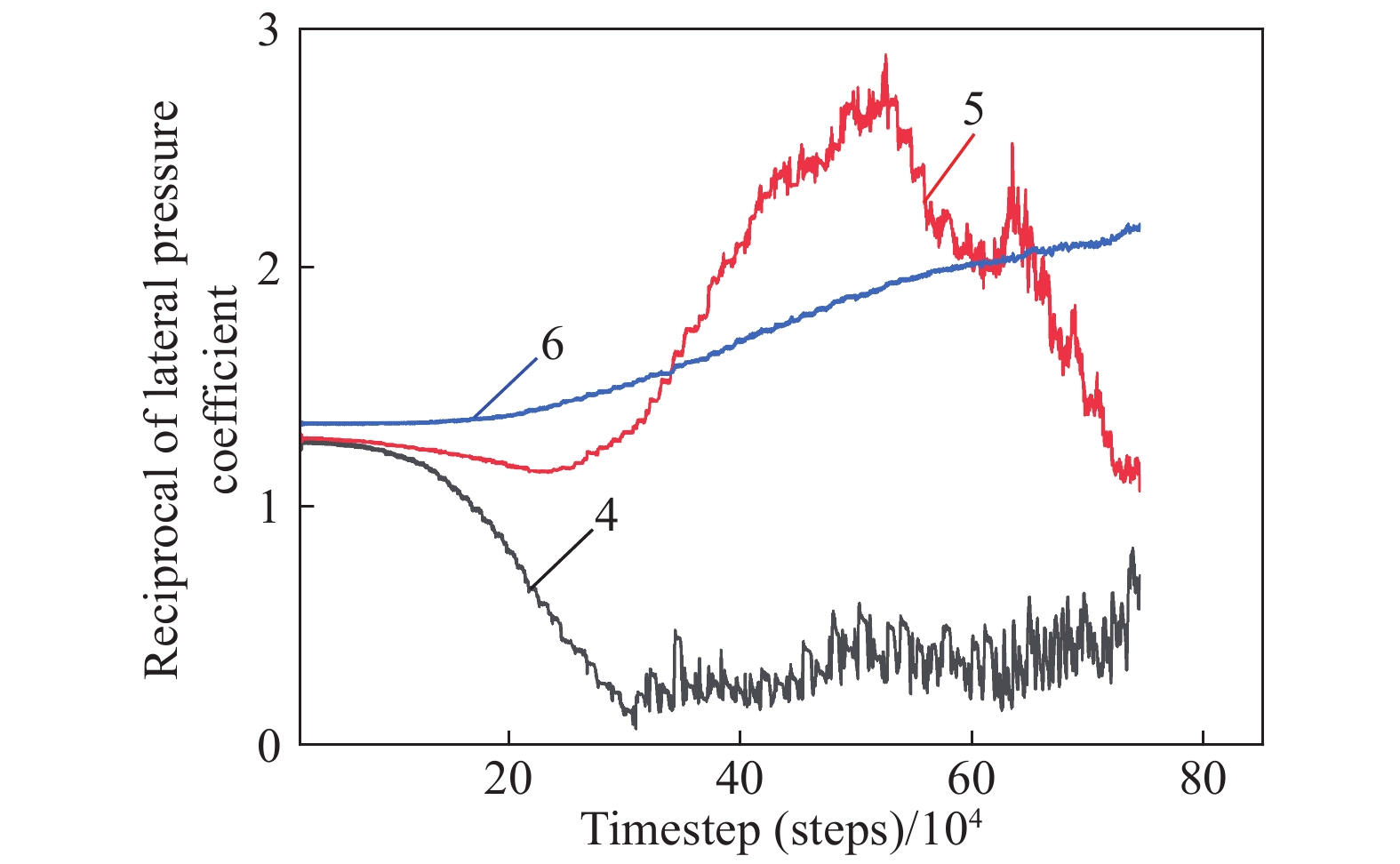
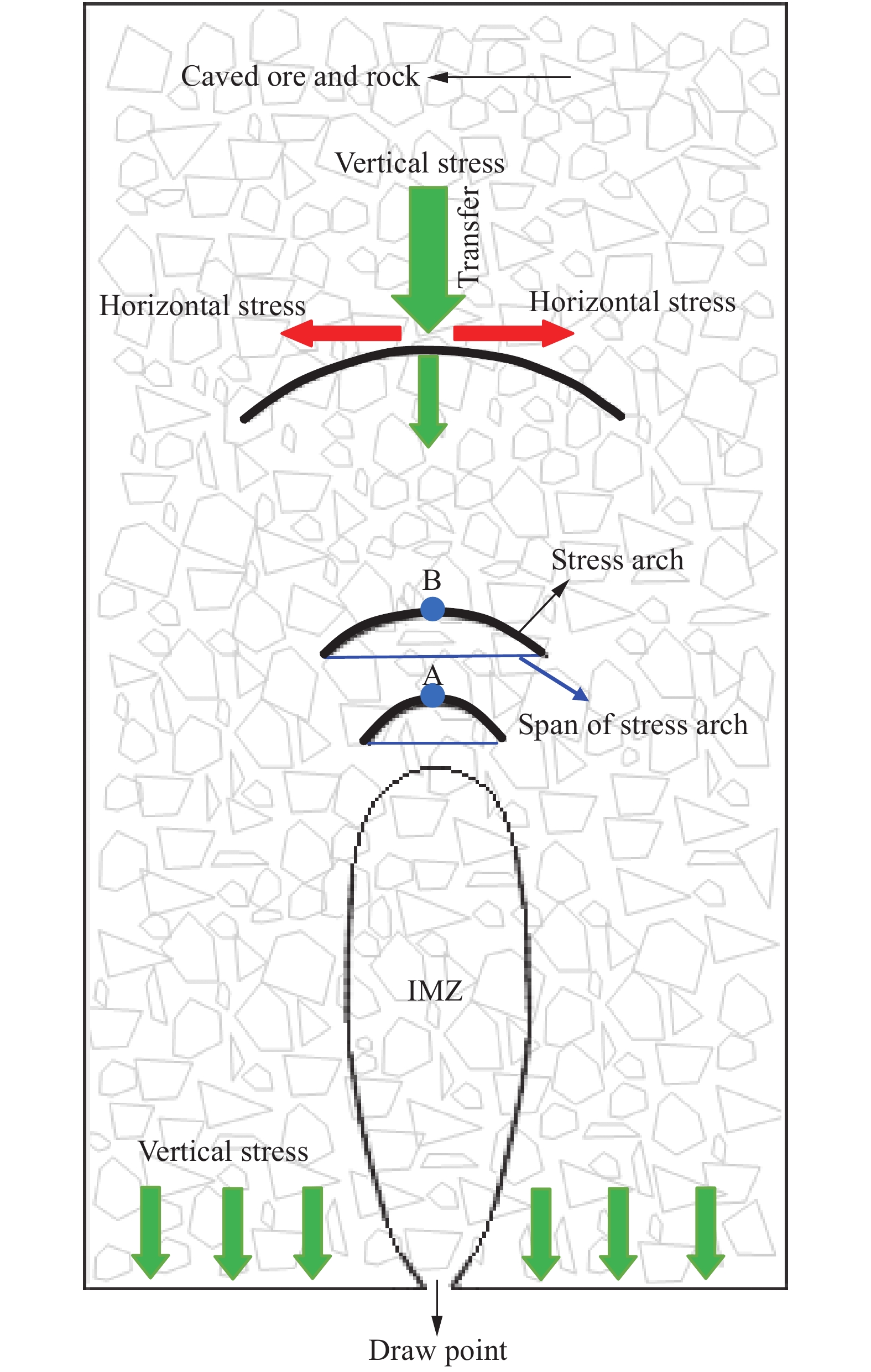
摘要: 為進一步揭示遠場條件下金屬礦山崩落礦巖運移演化機理，綜合利用物理試驗、數值模擬和理論分析等手段，構建單口放礦模型開展近?遠場崩落礦巖流動特性研究。首次基于離散元軟件PFC3D和剛性塊體模型構建放礦數值模型，并通過近場放礦物理試驗與模擬結果的對比分析，證明了剛性塊體模型在崩落礦巖流動特性研究中的可靠性與優越性。在此基礎上，對遠場條件下松動體形態變化規律、礦巖流動體系內的應力演化規律及其力學機理進行了量化研究。研究結果表明：1）近?遠場條件下的松動體形態變化均符合倒置水滴理論。在放礦初始階段，松動體最大寬度隨高度增大呈冪函數形式快速增加；隨后，松動體最大寬度隨高度增大而近似線性增加。2）崩落礦巖流動過程中存在明顯的應力拱效應。隨著礦巖散體松動范圍不斷擴大，松動體外圍一定范圍內的垂直應力均呈明顯下降趨勢，水平應力逐漸增大并在松動區域到達前出現激增現象；而松動體內的水平應力與垂直應力則急劇下降至較低水平。Abstract: The high mining costs of mines have led to the imbalance between the supply and demand of the total mineral resources in China and the dependence on imports to a large extent. Therefore, it is of great significance to expand the mining scale of mineral resources and reduce the mining costs to improve the self-sufficiency rate of mineral resources and strengthen social support and economic development in China. The caving mining method, especially the block caving method, has the following two main characteristics: one is that caved ores, surrounded by overlying rocks, are drawn from the drawpoint and the other one is that ground pressure is managed by filling goaf with overlying rocks. It is a low-cost and efficient large-scale underground mining method and has been widely used in metal mines around the world. To further reveal the far-field field migration and evolution mechanism of caved ore and rock in metal mine, through physical test, numerical simulation, and theoretical analysis, isolated-drawpoint draw models were constructed to study the flow characteristics of near/far-field flow characteristics of caved ore and rock. Based on the discrete element software PFC3D and rigid block model, the numerical draw model was constructed for the first time. The reliability and superiority of the rigid block model in the study of flow characteristics of caved ore and rock were proved by comparative analysis between near-field physical draw test results and simulated results. Moreover, the variation law of the IMZ (Isolated Movement Zone), the stress evolution law and its mechanical mechanism in the particle flow system under far-field conditions were quantitatively studied. The key research results prove that: 1) The shapes of IMZ under near/far-field conditions conform to the upside-down drop shape theory. In the initial draw stage, the maximum width of IMZ increases rapidly with the increase of height in the form of power function; while in the following draw stage, the maximum width of IMZ increases almost linearly with the height increase. 2) There is an obvious stress arch effect during the flow of caved ore and rock. With the range expansion of the caved ore and rock, the vertical stress in a certain range outside the IMZ decreases obviously, while the horizontal stress gradually increases and surges before the arrival of IMZ. Furthermore, the horizontal and vertical stresses within the IMZ drop sharply to a lower level.
-
Key words:
- draw /
- near/far-field condition /
- caved ore and rock /
- flow characteristics /
- rigid block model
-
圖 1 三維放礦物理與數值模型。（a）放礦物理試驗平臺；（b）放礦數值模型
Figure 1. 3D physical and numerical draw models: (a) physical draw test platform; (b) numerical draw model
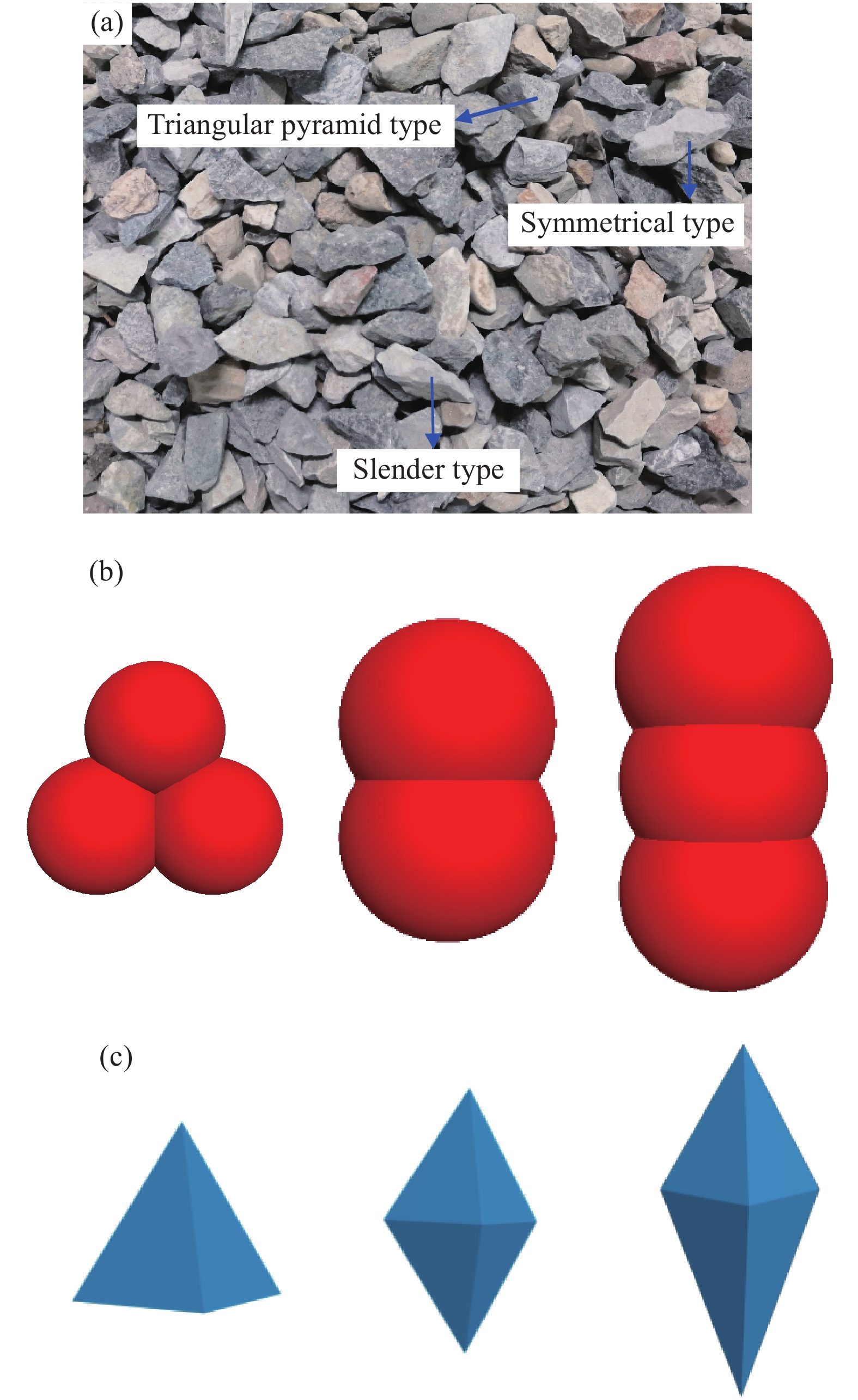
圖 2 物理與數值試驗中的三維顆粒形狀。（a）物理試驗中存在的顆粒形狀；（b）過往數值模擬中選用的顆粒形狀；（c）本次數值模擬中選用的顆粒形狀
Figure 2. 3D particle shapes used in physical and numerical draw tests: (a) particle shapes in the physical test; (b) particle shapes used in previous numerical simulations; (c) particle shapes used in these numerical simulations
圖 3 物理與數值試驗中的顆粒級配曲線
Figure 3. Particle size distribution curves in physical and numerical draw tests
圖 4 篩分后所得不同粒徑的石灰石散體。（a）3～8 mm；（b）8～16 mm；（c）16～25 mm；（d）25～45 mm
Figure 4. Limestone particles with different sizes after sieving: (a) 3?8 mm; (b) 8?16 mm; (c) 16?25 mm; (d) 25?45 mm
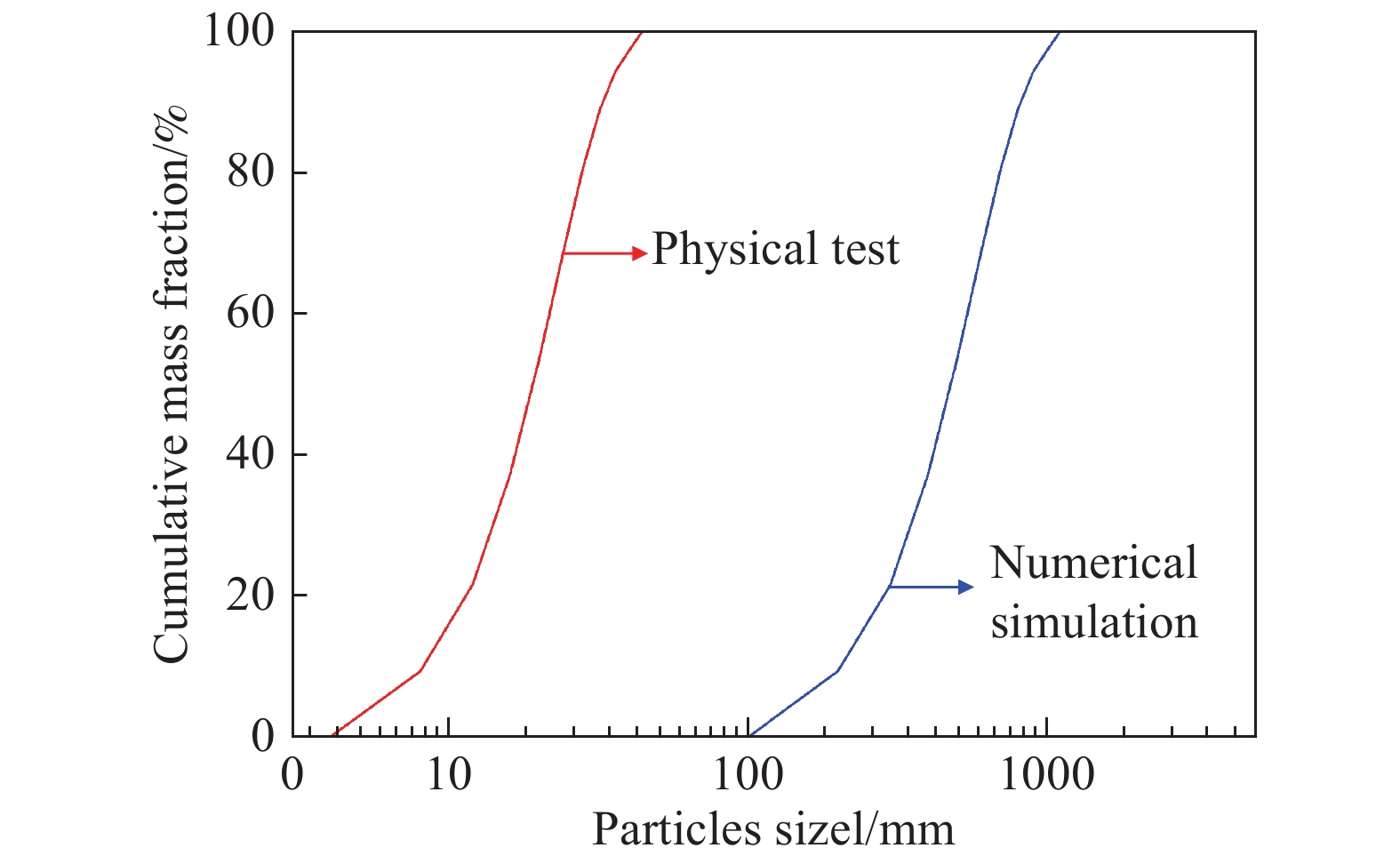
圖 6 放礦物理與數值試驗中的放出體與松動體形態縱剖面圖。（a）物理試驗中的放出體；（b）數值模擬中高度50 m的放出體；（c）數值模擬中高度50 m的松動體
Figure 6. Longitudinal profiles of the IEZ’s and IMZ’s shapes in physical and numerical draw tests: (a) IEZ in the physical test; (b) IEZ with a height of 50 m in numerical simulation; (c) IMZ with a height of 50 m in numerical simulation
圖 7 放出體、松動體高度與最大半徑關系的近場放礦物理與數值試驗結果對比
Figure 7. Comparison of relationship between the height and maximal radius of IEZ/IMZ in near-field physical and numerical draw tests
圖 8 遠場放礦數值模型縱剖面圖和應力測量域布設
Figure 8. Longitudinal profile of the far-field numerical draw model and layout of stress measurement regions
圖 9 松動體高度與最大半徑關系的遠場放礦數值模擬數據和理論曲線對比
Figure 9. Comparison between the data of far-field numerical draw test and theoretic curve for the relationship between the height and maximal radius of IMZ
圖 10 第1、4、5、6、7號測量域內的垂直應力變化過程
Figure 10. Variations of vertical stresses within measurement regions Nos. 1, 4, 5, 6, and 7
圖 11 第1、4、7號測量域內的水平應力變化過程
Figure 11. Variations of horizontal stresses within measurement regions Nos. 4, 5, and 6
圖 12 第4、5、6號測量域內側壓系數倒數的變化過程
Figure 12. Variations of reciprocal of the lateral pressure within measurement regions Nos. 4, 5, and 6
圖 13 礦巖顆粒流動體系內應力拱和應力轉移示意圖
Figure 13. Schematic of stress arch and stress transfer within the particle flow system of caved ore and rock
表 1 墻體及剛性塊體細觀力學參數
Table 1. Meso-mechanical parameters of walls and rigid blocks
Walls Rigid blocks Normal stiffness/
(N·m?1)Shear stiffness/
(N·m?1)Friction
coefficientNormal stiffness/
(N·m?1)Shear stiffness/
(N·m?1)Density/
(kg·m?3)Friction
coefficient5×107 5×107 0.50 3×107 3×107 2620 0.50 
下載: 導出CSV
259luxu-164<th id="5nh9l"></th> <strike id="5nh9l"></strike> <th id="5nh9l"><noframes id="5nh9l"><th id="5nh9l"></th> <strike id="5nh9l"></strike> <th id="5nh9l"><noframes id="5nh9l"> <th id="5nh9l"></th> <strike id="5nh9l"><noframes id="5nh9l"><span id="5nh9l"></span> <span id="5nh9l"><noframes id="5nh9l"><span id="5nh9l"></span> <strike id="5nh9l"><noframes id="5nh9l"><strike id="5nh9l"></strike> <span id="5nh9l"><noframes id="5nh9l"> <span id="5nh9l"><noframes id="5nh9l"> <span id="5nh9l"></span> <span id="5nh9l"><video id="5nh9l"></video></span> <th id="5nh9l"><noframes id="5nh9l"><th id="5nh9l"></th> -
參考文獻
[1] Shen N S, Gu X C, Yin S H. Technology status of block caving method at home and abroad. Min Technol, 2009, 9(4): 1 doi: 10.3969/j.issn.1671-2900.2009.04.001沈南山, 顧曉春, 尹升華. 國內外自然崩落采礦法技術現狀. 采礦技術, 2009, 9(4):1 doi: 10.3969/j.issn.1671-2900.2009.04.001 [2] Chitombo G P. Cave mining: 16 years after Laubscher’s 1994 paper 'Cave mining?state of the art'. Min Technol, 2010, 119(3): 132 doi: 10.1179/174328610X12820409992255 [3] Pierce M E. A Model for Gravity Flow of Fragmented Rock in Block Caving Mines[Dissertation]. Brisbane: The University of Queensland, 2010 [4] Wang H C. Ore Drawing. Beijing: Metallurgical Industry Press, 1982王漢昌. 放礦學. 北京: 冶金工業出版社, 1982 [5] Li R F, Guo J P. Quasi-ellipsoid Drawing Theory and Verification of Drawing. Beijing: Metallurgical Industry Press, 2016李榮福, 郭進平. 類橢球體放礦理論及放礦理論檢驗. 北京: 冶金工業出版社, 2016 [6] Ren F Y. Stochastic Medium Theory for Ore Drawing and Its Application. Beijing: Metallurgical Industry Press, 1994任鳳玉. 隨機介質放礦理論及其應用. 北京: 冶金工業出版社, 1994 [7] Fr?str?m J. Examination of Equivalent Model Materials for Development and Design of Sublevel Caving[Dissertation]. Stockholm: Royal Institute of Technology, 1970 [8] Jin A B, Sun H, Wu S C, et al. Confirmation of the upside-down drop shape theory in gravity flow and development of a new empirical equation to calculate the shape. Int J Rock Mech Min Sci, 2017, 92: 91 doi: 10.1016/j.ijrmms.2016.12.005 [9] ?ssr R K. Gravity flow of granular materials in hoppers and bins. Int J Rock Mech Min Sci Geomech Abs, 1965, 2(1): 25 doi: 10.1016/0148-9062(65)90020-3 [10] ?ssr R K. Gravity flow of granular materials in hoppers and bins in mines—Ⅱ. Coarse material. Int J Rock Mech Min Sci Geomech Abs, 1965, 2(3): 277 doi: 10.1016/0148-9062(65)90029-X [11] Janelid I, Kvapli R. Sublevel caving. Int J Rock Mech Min Sci Geomech Abs, 1966, 3(2): 129 doi: 10.1016/0148-9062(66)90004-0 [12] Laubscher D H. Block Cave Manual, Design Topic: Drawpoint Spacing and Draw Control[Dissertation]. Brisbane: The University of Queensland, 2000 [13] Power G R. Modelling Granular Flow in Caving Mines: Large Scale Physical Modelling and Full Scale Experiments [Dissertation]. Brisbane: The University of Queensland, 2004 [14] Castro R, Trueman R, Halim A. A study of isolated draw zones in block caving mines by means of a large 3D physical model. Int J Rock Mech Min Sci, 2007, 44(6): 860 doi: 10.1016/j.ijrmms.2007.01.001 [15] Tao G Q, Yang S J, Feng Y F. Experimental research on granular flow characters of caved ore and rock. Rock Soil Mech, 2009, 30(10): 2950 doi: 10.3969/j.issn.1000-7598.2009.10.010陶干強, 楊仕教, 任鳳玉. 崩落礦巖散粒體流動性能試驗研究. 巖土力學, 2009, 30(10):2950 doi: 10.3969/j.issn.1000-7598.2009.10.010 [16] Wang H J, Ying S H, Wu A X, et al. Experimental study of the factors affecting the ore flow mechanism during block caving. J China Univ Min Technol, 2010, 39(5): 693王洪江, 尹升華, 吳愛祥, 等. 崩落礦巖流動特性及影響因素實驗研究. 中國礦業大學學報, 2010, 39(5):693 [17] Wang Y P, Yu J. Optimization of breaking interval in non-pillar sublevel caving mining. J Cent South Univ Sci Technol, 2014, 45(2): 603王云鵬, 余健. 無底柱分段崩落法崩礦步距的優化. 中南大學學報(自然科學版), 2014, 45(2):603 [18] Sao A L. Experimental research on mullock movement in the side drawing. Min Metall Eng, 2012, 32(3): 1 doi: 10.3969/j.issn.0253-6099.2012.03.001邵安林. 端部放礦廢石移動規律試驗研究. 礦冶工程, 2012, 32(3):1 doi: 10.3969/j.issn.0253-6099.2012.03.001 [19] Xu S, An L, Li Y H, et al. Optimization of caving space for different angles of end-wall during pillarless sublevel caving. J Northeast Univ Nat Sci, 2012, 33(1): 120徐帥, 安龍, 李元輝, 等. 無底柱分段崩落法多端壁傾角下崩礦步距優化. 東北大學學報(自然科學版), 2012, 33(1):120 [20] Castro R, Pineda M. The role of gravity flow in the design and planning of large sublevel stopes. J South Afr Inst Min Metall, 2015, 115(2): 113 doi: 10.17159/2411-9717/2015/v115n2a4 [21] Sun H, Jin A B, Gao Y T, et al. Experimental research on the expectation body theory and optimization of the rate of advance during ore breaking in side drawing. Chin J Eng, 2016, 38(9): 1197孫浩, 金愛兵, 高永濤, 等. 期望體理論的實驗研究及端部放礦崩礦步距優化. 工程科學學報, 2016, 38(9):1197 [22] Cundall P A, Strack O D L. A discrete numerical model for granular assemblies. Geotechnique, 1979, 29(1): 47 doi: 10.1680/geot.1979.29.1.47 [23] Zhu H C. PFC and application case of caving study. Chin J Rock Mech Eng, 2006, 25(9): 1927 doi: 10.3321/j.issn:1000-6915.2006.09.030朱煥春. PFC及其在礦山崩落開采研究中的應用. 巖石力學與工程學報, 2006, 25(9):1927 doi: 10.3321/j.issn:1000-6915.2006.09.030 [24] Hashim M H M. Particle Percolation in Block Caving Mines[Dissertation]. Sydney: The University of New South Wales, 2011 [25] Song Z Y, Wei W J, Zhang J W. Numerical investigation of effect of particle shape on isolated extracted zone (IEZ) in block caving. Arab J Geosci, 2018, 11(12): 310 doi: 10.1007/s12517-018-3669-1 [26] Hu J H, Guo F Z, Luo X W, et al. Simulation of ore flow behavior and optimization of discharge parameters for caving method in gently inclined medium thickness ore-body. J Cent South Univ Sci Technol, 2015, 46(5): 1772 doi: 10.11817/j.issn.1672-7207.2015.05.027胡建華, 郭福鐘, 羅先偉, 等. 緩傾斜中厚礦體崩落開采礦石流動規律仿真與放礦參數優化. 中南大學學報(自然科學版), 2015, 46(5):1772 doi: 10.11817/j.issn.1672-7207.2015.05.027 [27] Sun H, Jin A B, Gao Y T, et al. Influencing factors on the flow characteristics of an isolated extraction zone in caving mining. Chin J Eng, 2015, 37(9): 1111孫浩, 金愛兵, 高永濤, 等. 崩落法采礦中放出體流動特性的影響因素. 工程科學學報, 2015, 37(9):1111 [28] Sun H, Jin A B, Gao Y T, et al. Flow characteristics of caved ore and rock under complex boundary conditions. J Cent South Univ Sci Technol, 2015, 46(10): 3782 doi: 10.11817/j.issn.1672-7207.2015.10.031孫浩, 金愛兵, 高永濤, 等. 復雜邊界條件下崩落礦巖流動特性. 中南大學學報(自然科學版), 2015, 46(10):3782 doi: 10.11817/j.issn.1672-7207.2015.10.031 [29] Sun H, Jin A B, Gao Y T, et al. Research of the isolated extraction zone form and determination of optimal independent advance under different end wall angles. Chin J Eng, 2016, 38(2): 159孫浩, 金愛兵, 高永濤, 等. 不同端壁傾角條件下放出體形態研究及最優崩礦步距的確定. 工程科學學報, 2016, 38(2):159 [30] Castro R, Gómez R, Pineda M, et al. Experimental quantification of vertical stresses during gravity flow in block caving. Int J Rock Mech Min Sci, 2020, 127: 104237 doi: 10.1016/j.ijrmms.2020.104237 [31] Rafiee R, Ataei M, Khalookakaie R, et al. Numerical modeling of influence parameters in cavabililty of rock mass in block caving mines. Int J Rock Mech Min Sci, 2018, 105: 22 doi: 10.1016/j.ijrmms.2018.03.001 [32] Itasca Consulting Group Inc. PFC 6.0 documentation[EB/OL]. Itasca Consulting Group Inc (2019)[2020-07-11]. http://docs.itascacg.com/pfc600/pfc/docproject/index.html [33] Sun H, Jin A B, Elmo D, et al. A numerical based approach to calculate ore dilution rates using rolling resistance model and upside-down drop shape theory. Rock Mech Rock Eng, 2020, 53(10): 4639 doi: 10.1007/s00603-020-02180-6 [34] Sun H. Study on Migration and Evolution Mechanism of Caved Ore and Rock Based on the Particle Flow Theory[Dissertation]. Beijing: University of Science and Technology Beijing, 2019孫浩. 基于顆粒元理論的崩落礦巖運移演化機理研究[學位論文]. 北京: 北京科技大學, 2019 [35] Castro R L. Study of the Mechanisms of Gravity Flow for Block Caving[Dissertation]. Brisbane: University of Queensland, 2007 [36] Arévalo R, Maza D, Pugnaloni L A. Identification of arches in two-dimensional granular packings. Phys Rev E Stat Nonlin Soft Matter Phys, 2006, 74(2): 021303 doi: 10.1103/PhysRevE.74.021303 -
點擊查看大圖
計量
- 文章訪問數: 2116
- HTML全文瀏覽量: 756
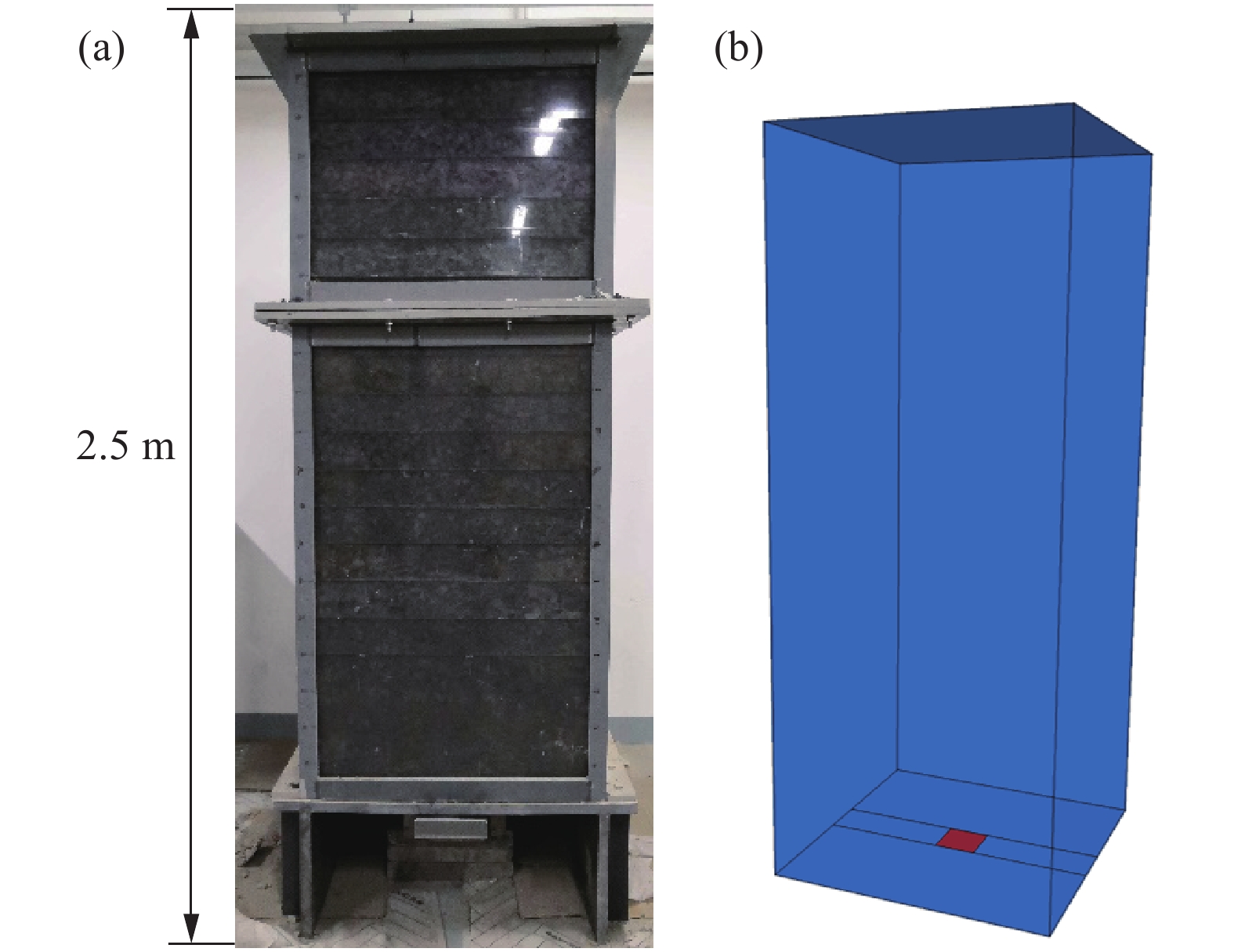
- PDF下載量: 50
- 被引次數: 0
