裂縫網絡支撐劑非均勻分布對開采動態規律的影響

朱維耀; 張啟濤; 岳明; 張燎源

doi:10.13374/j.issn2095-9389.2019.10.23.001

裂縫網絡支撐劑非均勻分布對開采動態規律的影響

doi: 10.13374/j.issn2095-9389.2019.10.23.001

1.
北京科技大學土木與資源工程學院，北京 100083
2.
中國石化勝利油田分公司石油工程技術研究院，東營 257000

基金項目: 國家科技重大專項資助項目(2017ZX05069-003)；教育部專項資金資助項目(FRF-TP-17-027A2)

詳細信息

通訊作者:
E-mail：weiyaook@sina.com

中圖分類號: TG357.12
計量
- 文章訪問數: 3627
- HTML全文瀏覽量: 960
- PDF下載量: 51
- 被引次數: 0
出版歷程
- 收稿日期: 2019-10-23
- 刊出日期: 2020-10-25

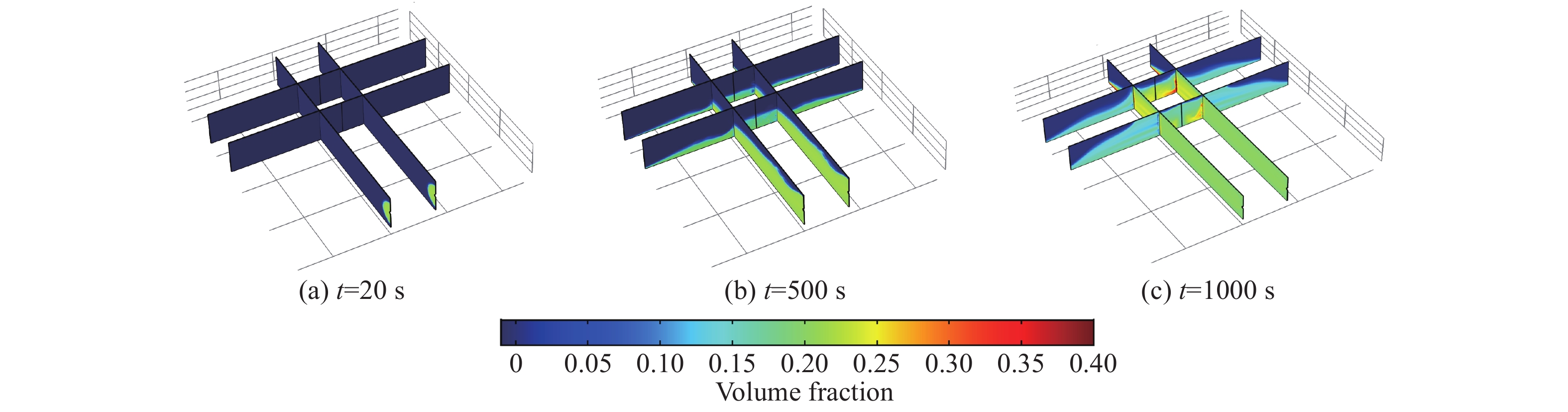
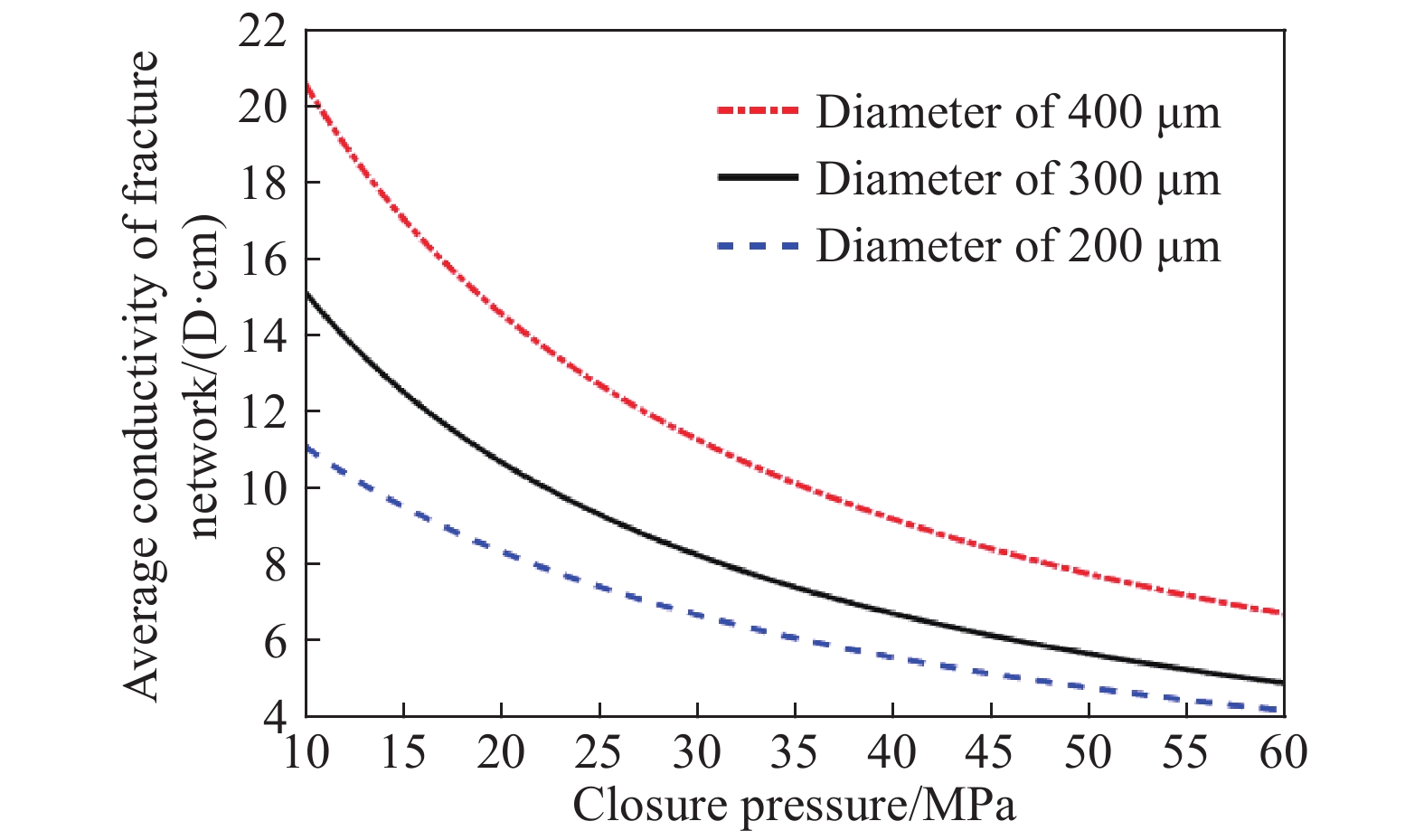
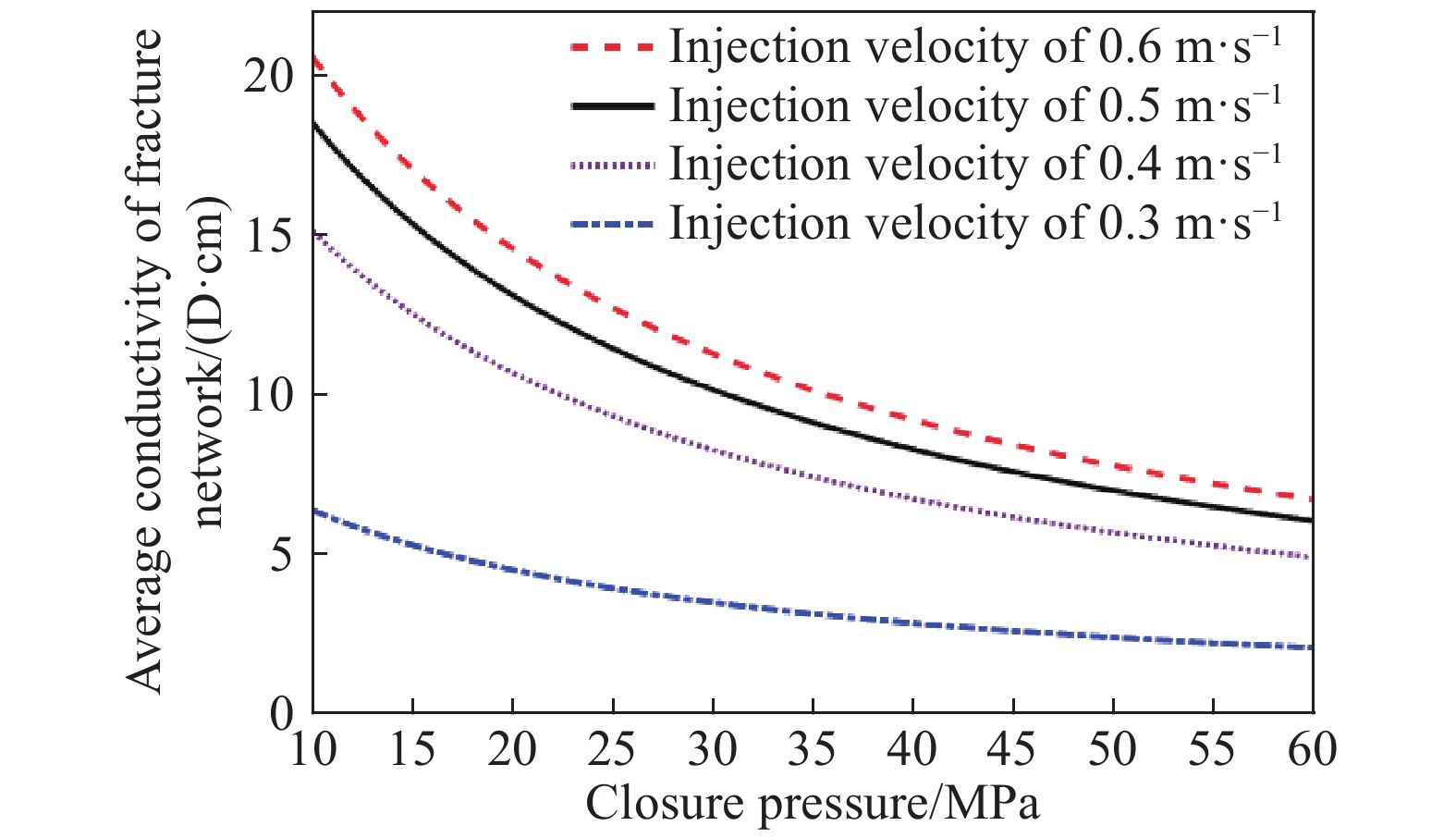
Effect of uneven distribution of proppant in fracture network on exploitation dynamic characteristics

1.
School of Civil and Resource Engineering, University of Science and Technology Beijing, Beijing 100083, China
2.
Research Institute of Petroleum Engineering, Shengli Oilfield Company, Dongying 257000, China

More Information

Corresponding author: E-mail: weiyaook@sina.com

摘要

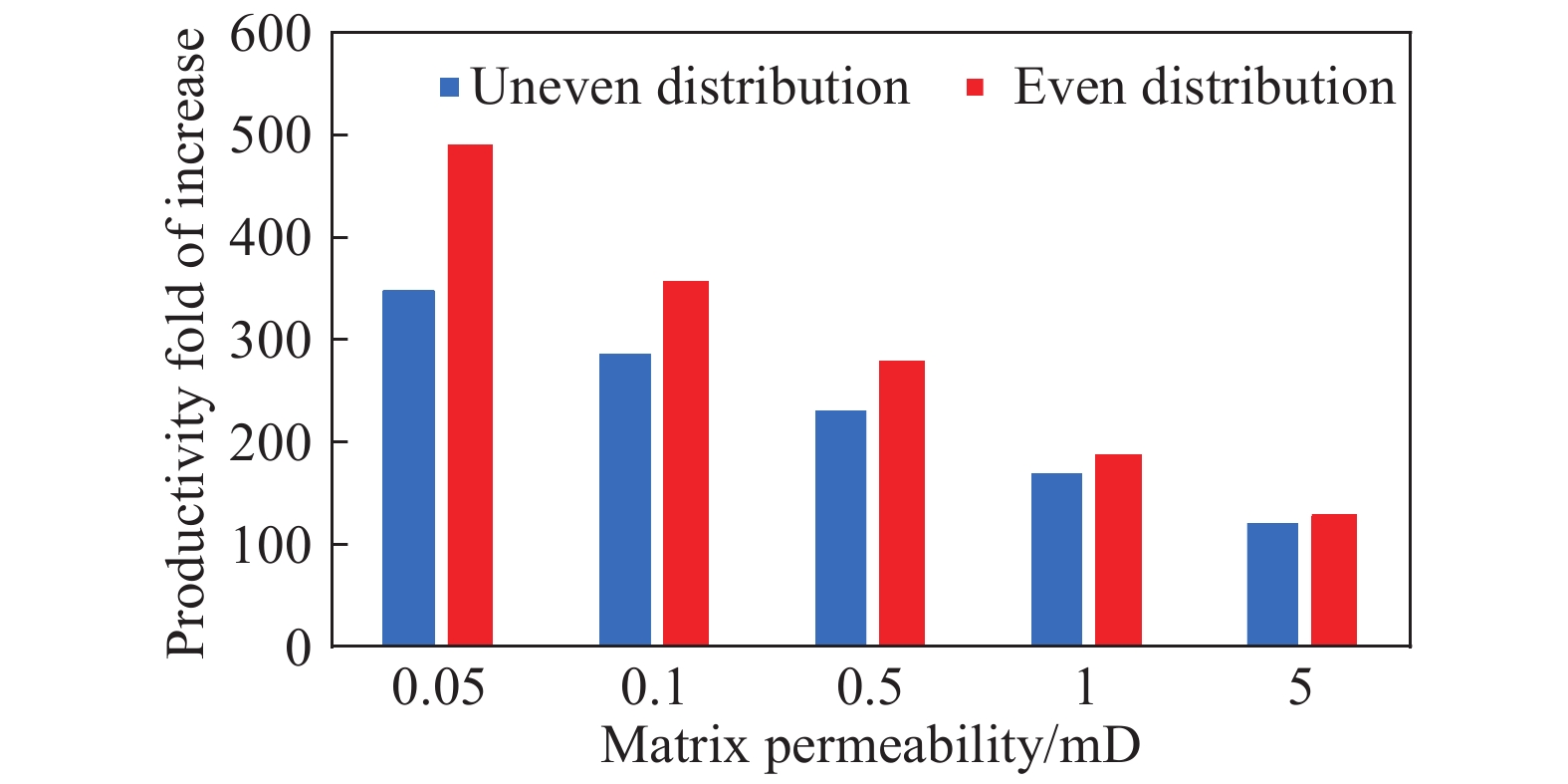
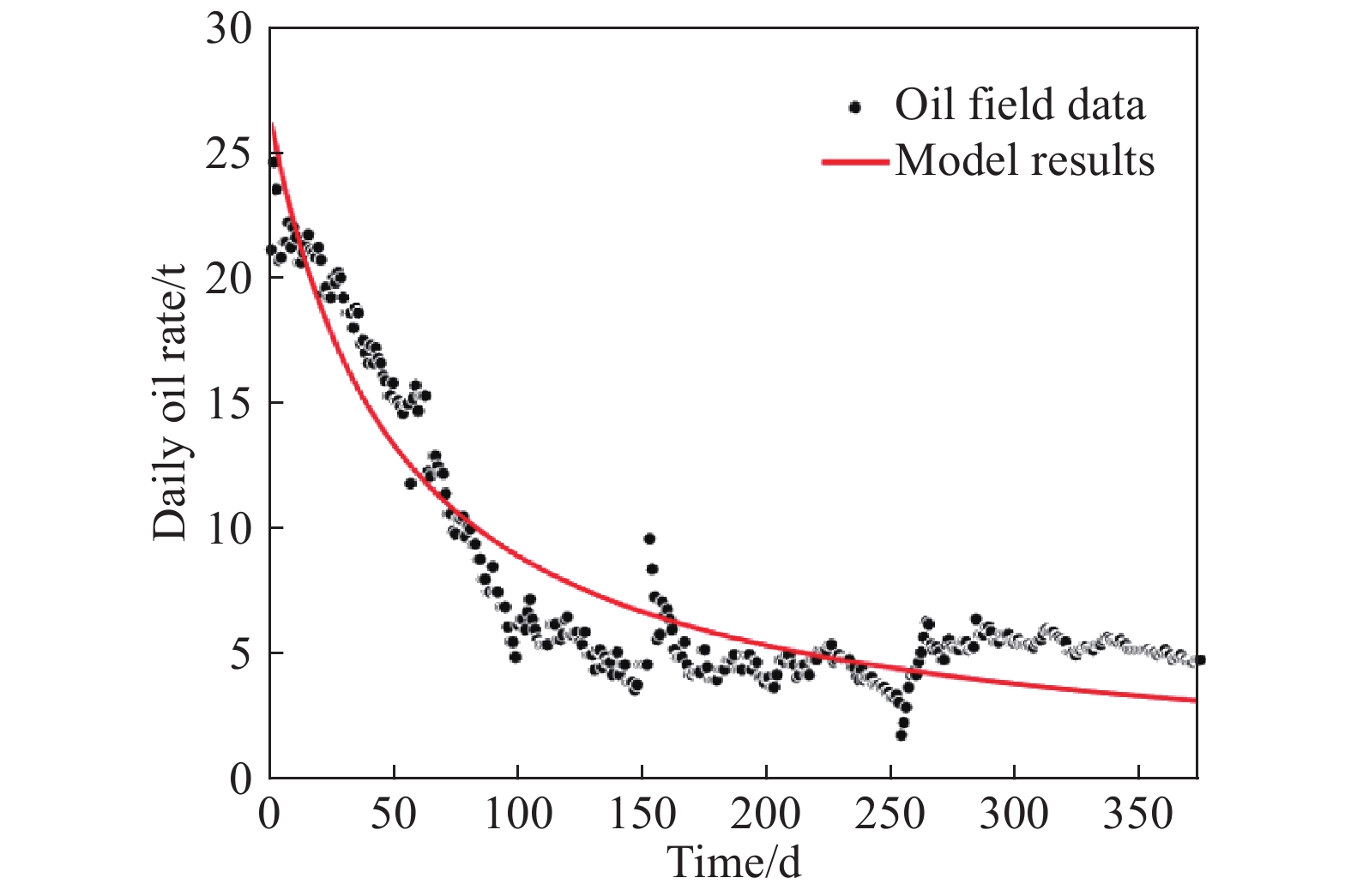
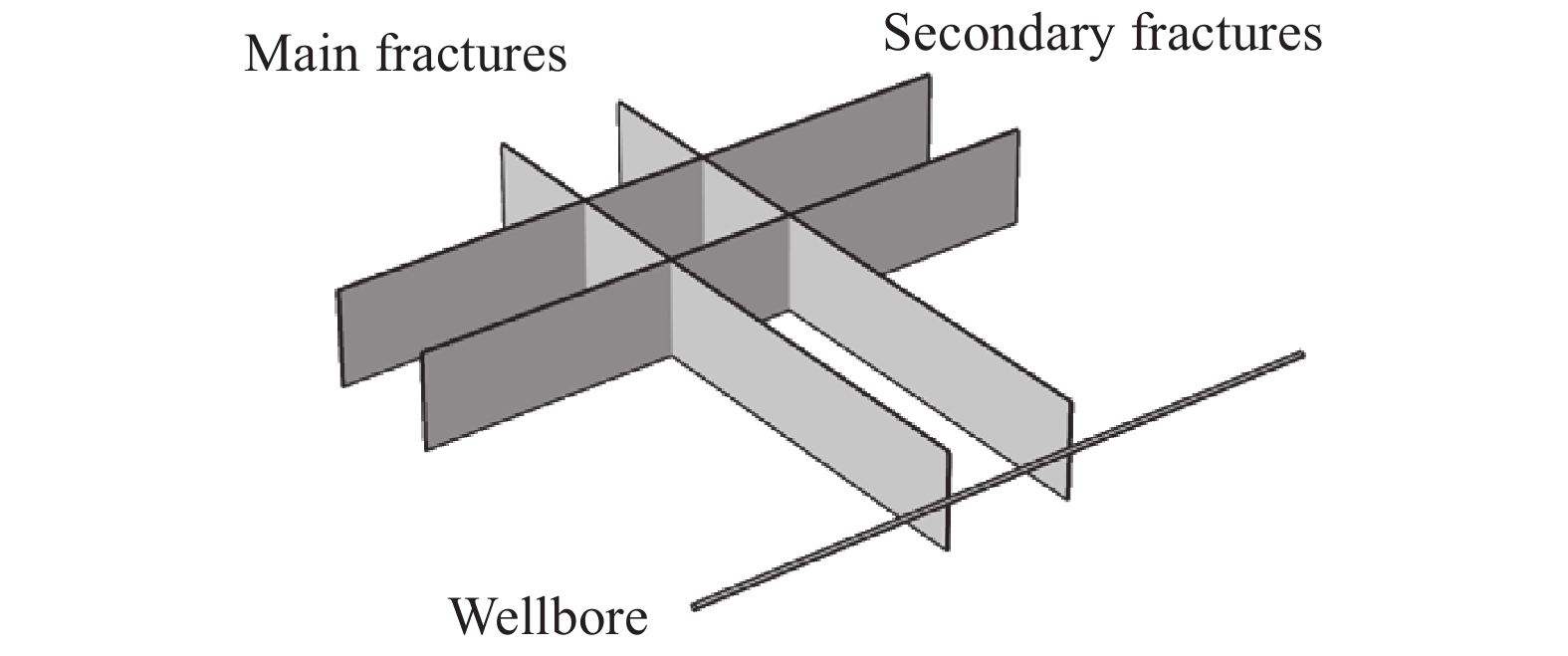
摘要: 水力壓裂過程中支撐劑的注入是為了防止地應力將已壓裂出的裂縫重新閉合。為了研究復雜裂縫網絡中支撐劑的運移分布規律，及支撐劑非均勻分布對開采動態規律的影響，基于作者之前提出的數個數學模型，建立了致密儲層壓裂注砂開發耦合計算模型。通過計算結果可以得知：裂縫網絡中，支撐劑會在裂縫交匯處大量堆積，砂堤高度高于縫網其他部分。次級裂縫中的支撐劑更多的處于懸浮狀態，且支撐劑沉降堆積高度相較于主裂縫小25%～50%，相互溝通的次級縫具有更高的支撐劑沉降程度。縫網中支撐劑非均勻分布對模擬計算結果具有較大影響，當儲層滲透率為0.05 mD時，忽略支撐劑非均勻分布計算出的產量高出實際值41.7%，因此在進行低滲透率儲層模擬時，支撐劑非均勻分布狀態不可忽略；當基質滲透率為5 mD時，產量計算差異在5%以內，此時不考慮支撐劑非均勻分布相對合理。
- 水平井 /
- 裂縫網絡 /
- 攜砂液 /
- 數值模擬 /
- 兩相流
Abstract: Proppant injection during hydraulic fracturing is to prevent the closure of hydraulic fractures. As a result, the distribution of proppant in the fracture impact the productivity to a great extent. In order to study the rule of proppant transportation and distribution in complex fracture network, and the influence of uneven proppant distribution on the exploitation dynamic characteristics, a full-coupled 3D finite element method calculation model for tight oil reservoir considering sand injection during hydraulic fracturing was established, based on several mathematical models proposed by the author in the past. In the model, a mixture model was utilized, which had advantages to deal with two-phase flow containing solid particles in dispersed phase, to simulate the proppant transportation process in fracture networks. Then, a tight oil reservoir model was established to evaluate the effect of the proppant distribution on the reservoir performance. The calculation results show that in the fracture network, proppant particles will accumulate at the fracture intersection, and the proppant concentration is higher than other parts of the fracture network. The height of proppant settlement dune in the secondary fracture is 25%–50% lower than that in the main fracture, and the communication of secondary fractures has enhanced the proppant settlement degree. Moreover, factors like injection velocity, proppant materials and proppant size are proved to have a strong relation to the average conductivity of fracture network, which could impact the fracture design considerably. Furthermore, the uneven distribution of proppant in fracture network has a great influence on the simulation results. When the reservoir permeability reaches 0.05 mD, the calculation results show that the height, without considering the uneven distribution, of proppant settlement is 41.7% higher than the actual value. Therefore, the uneven distribution of proppant cannot be ignored in the simulation of low permeability reservoir. However, when the matrix permeability is 5 mD, the difference between the actual and simulated result will be within 5%. Thus, it is reasonable to neglect the uneven distribution of proppant in estimations.
- horizontal well /
- fracture network /
- proppant-laden fluid /
- numerical simulation /
- two phase flow

HTML全文

圖 1 裂縫網絡幾何模型示意圖

Figure 1. Illustration of fracture network model

下載: 全尺寸圖片幻燈片

圖 2 裂縫網絡對稱簡化示意圖

Figure 2. Symmetric fracture network model

下載: 全尺寸圖片幻燈片

圖 3 裂縫網絡鋪砂過程示意圖

Figure 3. Illustration of proppant-laden fluid injection process

下載: 全尺寸圖片幻燈片

圖 4 支撐劑粒徑對縫網整體導流能力的影響

Figure 4. Effect of proppant diameter on fracture network conductivity

下載: 全尺寸圖片幻燈片

圖 5 支撐劑材料對縫網整體導流能力的影響

Figure 5. Effect of proppant materials on fracture network conductivity

下載: 全尺寸圖片幻燈片

圖 6 攜砂液注入速度對縫網整體導流能力的影響

Figure 6. Effect of proppant-laden fluid injection velocity on fracture network conductivity

下載: 全尺寸圖片幻燈片

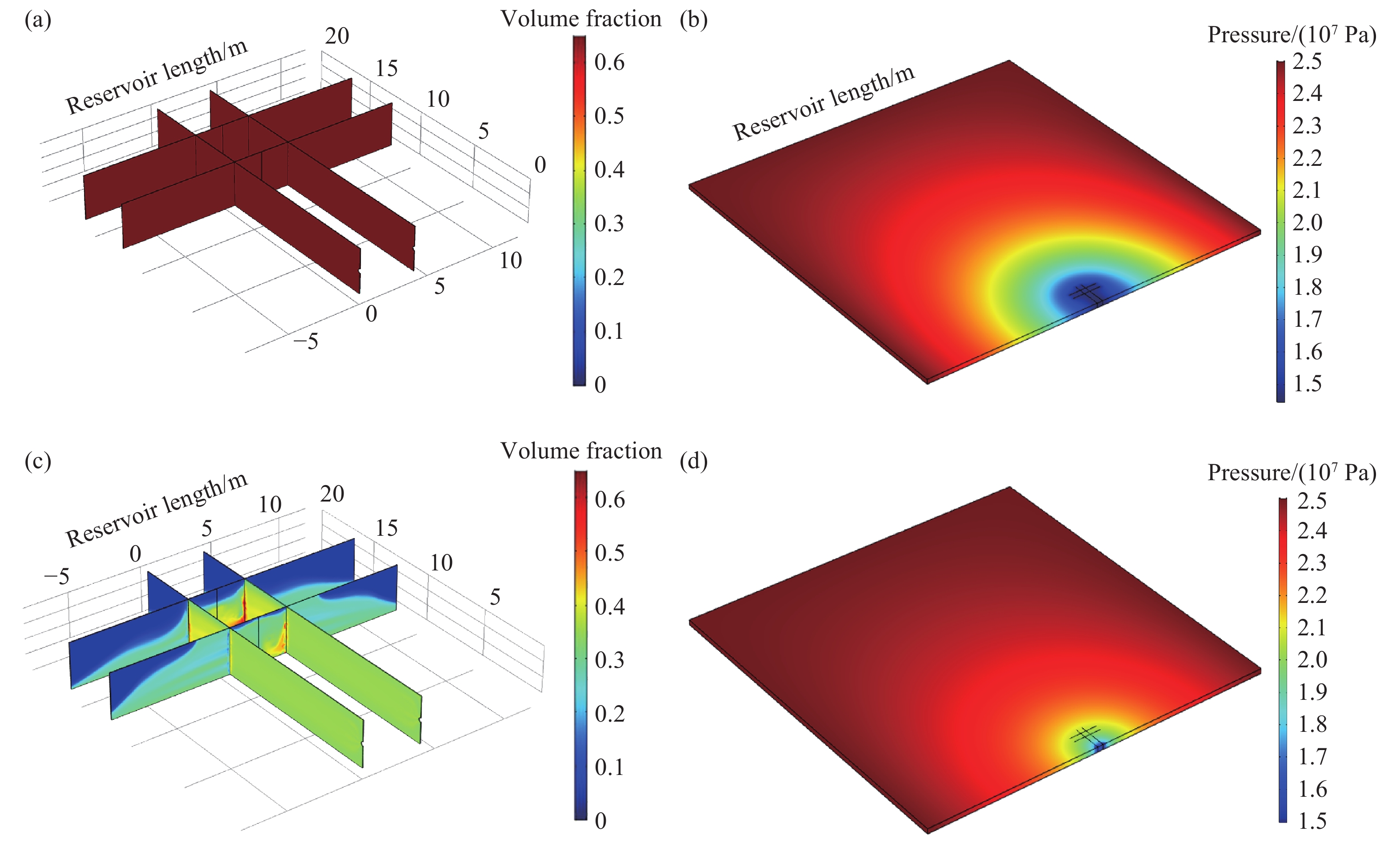
圖 7 裂縫網絡內支撐劑理想均勻分布與不均勻分布條件下的致密儲層開發300 d壓力場對比。（a）支撐劑均勻分布狀態；（b）基于支撐劑均勻分布的儲層壓力場分布；（c）支撐劑不均勻分布；（d）基于支撐劑不均勻分布的儲層壓力場分布

Figure 7. Comparison between pressure distribution based on proppant idealized and uneven distribution at 300 days in a tight oil reservoir: (a) idealized proppant distribution; (b) pressure distribution with even proppant distribution; (c) uneven proppant distribution; (d) pressure distribution with uneven proppant distribution

下載: 全尺寸圖片幻燈片

圖 8 基質滲透率對計算產量差異的影響

Figure 8. Effect of matrix permeability on difference in calculated productivity

下載: 全尺寸圖片幻燈片

圖 9 耦合計算模型結果與實際生產數據對比

Figure 9. Comparison of oil rate between coupling model and oil field data

下載: 全尺寸圖片幻燈片

表 1 基本計算參數

Table 1. Basic calculation parameters

Parameter	Value	Parameters	Value
Proppant density/(kg·m^?3)	2600	Proppant diameter/mm	0.3
Injection velocity/(m·s^?1)	0.4	Proppant volume fraction	0.3
Fluid density/(kg·m^?3)	1100	Fluid viscosity/(mPa?s)	5

下載: 導出CSV

表 2 基本計算參數

Table 2. Basic calculation parameters

Parameters	Value	Parameters	Value
Reservoir length/m	250	Reservoir width/m	250
Reservoir height/m	10	Permeability of matrix/mD	0.5
TPG in matrix/(MPa·m^?1)	0.05	Porosity of matrix/%	9.14
Wellbore pressure/MPa	15	Initial reservoir pressure/MPa	25
Note: TPG—Threshold pressure gradient.

下載: 導出CSV

259luxu-164

參考文獻(28)

[1]	Huang Y Z, Huang J L, Ge C M, et al. A key factor promoting rapid development of shale gas in America: technical progress. Nat Gas Ind, 2009, 29(5): 7 doi: 10.3787/j.issn.1000-0976.2009.05.002 黃玉珍, 黃金亮, 葛春梅, 等. 技術進步是推動美國頁巖氣快速發展的關鍵. 天然氣工業, 2009, 29(5):7 doi: 10.3787/j.issn.1000-0976.2009.05.002
[2]	Zhu W Y, Yue M, Liu Y F, et al. Research progress on tight oil exploration in China. Chin J Eng, 2019, 41(9): 1103 朱維耀, 岳明, 劉昀楓, 等. 中國致密油藏開發理論研究進展. 工程科學學報, 2019, 41(9):1103
[3]	Zhu W Y, Ma D X, Qi Q, et al. Multi-zone coupling productivity of horizontal well fracturing with complex fracture networks in shale gas reservoirs. Nat Gas Ind, 2017, 37(7): 60 doi: 10.3787/j.issn.1000-0976.2017.07.009 朱維耀, 馬東旭, 亓倩, 等. 復雜縫網頁巖壓裂水平井多區耦合產能分析. 天然氣工業, 2017, 37(7):60 doi: 10.3787/j.issn.1000-0976.2017.07.009
[4]	Tang Y, Tang X, Wang G Y, et al. Summary of hydraulic fracturing technology in shale gas development. Geol Bull China, 2011, 30(2-3): 393 唐穎, 唐玄, 王廣源, 等. 頁巖氣開發水力壓裂技術綜述. 地質通報, 2011, 30(2-3):393
[5]	Liu D H, Xiao J L, Guan F J. Current situation and research direction of shale gas development. J Oil Gas Technol, 2011, 33(1): 119 doi: 10.3969/j.issn.1000-9752.2011.01.027 劉德華, 肖佳林, 關富佳. 頁巖氣開發技術現狀及研究方向. 石油天然氣學報, 2011, 33(1):119 doi: 10.3969/j.issn.1000-9752.2011.01.027
[6]	Economides M J, Nolte K G. Reservoir Stimulation. Schlumerger Educational Services, 1989
[7]	Osiptsov A A. Fluid mechanics of hydraulic fracturing: a review. J Pet Sci Eng, 2017, 156: 513 doi: 10.1016/j.petrol.2017.05.019
[8]	Patankar N A, Joseph D D, Wang J, et al. Power law correlations for sediment transport in pressure driven channel flows. Int J Multiphase Flow, 2002, 28(8): 1269 doi: 10.1016/S0301-9322(02)00030-7
[9]	Wen Q Z, Jin X C, Shah S N, et al. Experimental investigation of propped fracture network conductivity in naturally fractured shale reservoirs // SPE Annual Technical Conference and Exhibition. New Orleans, 2013: 166474
[10]	Wen Q Z, Zhai H L, Luo M L, et al. Study on proppant settlement and transport rule in shale gas fracturing. Petrol Geol Recovery Efficiency, 2012, 19(6): 104 doi: 10.3969/j.issn.1009-9603.2012.06.025 溫慶志, 翟恒立, 羅明良, 等. 頁巖氣藏壓裂支撐劑沉降及運移規律實驗研究. 油氣地質與采收率, 2012, 19(6):104 doi: 10.3969/j.issn.1009-9603.2012.06.025
[11]	Wen Q Z, Luo M L, Li J N, et al. Principle of proppant settlement in fracture. Pet Geol Recovery Efficiency, 2009, 16(3): 100 doi: 10.3969/j.issn.1009-9603.2009.03.033 溫慶志, 羅明良, 李加娜, 等. 壓裂支撐劑在裂縫中的沉降規律. 油氣地質與采收率, 2009, 16(3):100 doi: 10.3969/j.issn.1009-9603.2009.03.033
[12]	Li J H, Zhu W Y, Yue M, et al. Numerical simulation of proppant-laden fluid flow in single fracture and branch fractures of horizontal well. Sci Technol Eng, 2018, 18(22): 38 doi: 10.3969/j.issn.1671-1815.2018.22.005 李劍輝, 朱維耀, 岳明, 等. 水平井壓裂單縫和多分支縫中攜砂液流動規律數值模擬. 科學技術與工程, 2018, 18(22):38 doi: 10.3969/j.issn.1671-1815.2018.22.005
[13]	Zhang L Y, Zhai H L, Lu N N, et al. Experimental study on proppant gathered settlement in non-Newtonian fracturing fluid. Sci Technol Eng, 2013, 13(34): 10142 doi: 10.3969/j.issn.1671-1815.2013.34.007 張潦源, 翟恒立, 盧娜娜, 等. 非牛頓壓裂液中支撐劑聚集沉降規律實驗研究. 科學技術與工程, 2013, 13(34):10142 doi: 10.3969/j.issn.1671-1815.2013.34.007
[14]	Barree R D, Conway M W. Experimental and numerical modeling of convective proppant transport. J Pet Technol, 1995, 47(3): 28564
[15]	Cipolla C L, Warpinski N R, Mayerhofer M J. Hydraulic fracture complexity: diagnosis, remediation, and explotation // SPE Asia Pacific Oil and Gas Conference and Exhibition. Perth, 2008
[16]	Maxwell S C, Urbancic T I, Steinsberger N P, et al. Microseismic imaging of hydraulic fracture complexity in the barnett shale // SPE Annual Technical Conference and Exhibition. San Antonio, 2002
[17]	Wen Q Z, Duan X F, Zhan Y P, et al. Study on settlement and migration law of proppant in complex fracture network. J Xi’an Shiyou Univ Nat Sci, 2016, 31(1): 79 溫慶志, 段曉飛, 戰永平, 等. 支撐劑在復雜縫網中的沉降運移規律研究. 西安石油大學學報: 自然科學版, 2016, 31(1):79
[18]	Li Y. Study on Proppants Settlement Law in Fracture Network of Volume Fracturing[Dissertation]. Qingdao: China University of Petroleum (East China), 2015 李楊. 體積壓裂復雜縫網支撐劑沉降規律研究[學位論文]. 青島: 中國石油大學(華東), 2015
[19]	Sahai R, Miskimins J L, Olson K E. Laboratory results of proppant transport in complex fracture systems // SPE Hydraulic Fracturing Technology Conference. Woodlands, 2014: 35
[20]	Zhu W Y, Liu Q, Yue M, et al. Calculation of fracture conductivity considering proppant influence and simulation of proppant transport in fracture. Chem Eng Oil Gas, 2019, 48(2): 75 doi: 10.3969/j.issn.1007-3426.2019.02.013 朱維耀, 劉青, 岳明, 等. 考慮支撐劑裂縫導流能力計算及縫內支撐劑運移模擬. 石油與天然氣化工, 2019, 48(2):75 doi: 10.3969/j.issn.1007-3426.2019.02.013
[21]	Liu W C, Zhang Q T, Zhu W Y. Numerical simulation of multi-stage fractured horizontal well in low-permeable oil reservoir with threshold pressure gradient with moving boundary. J Pet Sci Eng, 2019, 178: 1112 doi: 10.1016/j.petrol.2019.04.033
[22]	Ishii M, Hibiki T. Thermo-Fluid Dynamics of Two-Phase Flow. Springer Science & Business Media, 2010
[23]	Wu Y L, Liu S H, Dou H S, et al. Simulations of unsteady cavitating turbulent flow in a Francis turbine using the RANS method and the improved mixture model of two-phase flows. Eng Comput, 2011, 27(3): 235 doi: 10.1007/s00366-010-0194-6
[24]	Aminfar H, Mohammadpourfard M, Mohseni F. Two-phase mixture model simulation of the hydro-thermal behavior of an electrical conductive ferrofluid in the presence of magnetic fields. J Magn Magn Mater, 2012, 324(5): 830 doi: 10.1016/j.jmmm.2011.09.028
[25]	Krieger I M, Dougherty T J. A mechanism for non-Newtonian flow in suspensions of rigid spheres. Trans Soc Rheol, 1959, 3: 137 doi: 10.1122/1.548848
[26]	Warren J E, Root P J. The behavior of naturally fractured reservoirs. Soc Pet Eng J, 1963, 3(03): 245 doi: 10.2118/426-PA
[27]	Mayerhofer M J, Lolon E, Warpinski N R, et al. What is stimulated reservoir volume. SPE Prod Oper, 2010, 25(1): 89
[28]	Kong B, Fathi E, Ameri S. Coupled 3-D numerical simulation of proppant distribution and hydraulic fracturing performance optimization in Marcellus shale reservoirs. Int J Coal Geol, 2015, 147-148: 35 doi: 10.1016/j.coal.2015.06.006